Latest Applications Open 2024:
The National Testing Agency (NTA) has officially released the Mathematics syllabus for JEE Main 2025, which is a crucial part of Paper 1. This paper comprises three core subjects: Physics, Chemistry, and Mathematics (PCM). The Mathematics section is integral to the examination and encompasses a broad range of topics essential for a comprehensive understanding of the subject.
The JEE Main 2025 Mathematics syllabus draws from the class 11th and 12th curricula of both CBSE and various State Boards. It includes key areas of study designed to evaluate a candidate’s proficiency in mathematical concepts and problem-solving skills. The syllabus is structured to cover a wide spectrum of mathematical disciplines, ensuring that students are well-prepared for the exam.
Candidates are advised to thoroughly review the syllabus as outlined by the NTA to ensure they cover all necessary topics. This preparation will help them tackle the examination more effectively, as it provides a detailed framework of what to expect in the Mathematics section of JEE Main 2025.
JEE Main 2025 Mathematics Syllabus – Released
JEE Main 2025 Mathematics Syllabus has been Released. Click to Download Syllabus PDF.
Other JEE Main Related Syllabus Link
| JEE Main Syllabus | JEE Main Physics Syllabus |
| JEE Main Chemistry Syllabus | JEE Main Preparation |
| JEE Main Eligibility | JEE Main Exam Pattern |
JEE Main Maths Syllabus 2025
The JEE Main 2025 Mathematics syllabus outlines the key topics and concepts that candidates need to master for Paper 1 of the exam. Based on the Class 11th and 12th CBSE and State Board curricula, this syllabus includes essential units in Algebra, Calculus, Coordinate Geometry, and more, aimed at assessing a comprehensive understanding of mathematical principles.
UNIT 1: SETS, RELATIONS, AND FUNCTIONS
This unit covers foundational concepts in set theory and functions. It begins with sets and their representation, including operations such as union, intersection, and complement, as well as their algebraic properties. Students will also explore the power set and understand how sets can be organized and manipulated.
The unit further delves into relations, defining different types such as equivalence relations and their properties. It examines functions, focusing on the concepts of one-to-one (injective), into (surjective), and onto (bijective) functions. Additionally, the composition of functions is studied, highlighting how functions can be combined to form new functions. This section is fundamental for building a solid base in mathematical reasoning and analysis.
UNIT 2: COMPLEX NUMBERS AND QUADRATIC EQUATIONS
This unit explores complex numbers and their properties. It begins with complex numbers as ordered pairs of real numbers, denoted as a+iba + iba+ib, and their graphical representation using the Argand diagram. The algebraic operations on complex numbers, including addition, subtraction, multiplication, and division, are covered, along with the concepts of modulus and argument (or amplitude).
The unit also addresses quadratic equations, both in the context of real and complex number systems. It examines the solutions of these equations, focusing on the relationships between roots and coefficients. Topics include the nature of roots, such as real or imaginary, and the process of forming quadratic equations given specific roots. Understanding these concepts is essential for solving polynomial equations and applying complex number theory in various mathematical contexts.
UNIT 3: MATRICES AND DETERMINANTS
Unit 3 covers fundamental concepts in matrices and determinants. It introduces the basics of matrices, including various types and algebraic operations. Key topics include the evaluation of determinants for matrices of order two and three and their applications such as calculating areas of triangles.
The unit also explores the adjoint of a matrix and methods for finding the inverse using determinants. Additionally, it addresses solving simultaneous linear equations in two or three variables through matrix methods, focusing on consistency tests and solution techniques.
UNIT 4: PERMUTATIONS AND COMBINATIONS
Unit 4 explores permutations and combinations, fundamental concepts in counting and arrangement. It covers the fundamental principle of counting, permutations (arrangements of objects) denoted as P(n,r)P(n, r)P(n,r), and combinations (selections of objects) denoted as C(n,r)C(n, r)C(n,r). The unit includes simple applications of these principles, emphasizing practical problem-solving in counting and arrangement scenarios.
UNIT 5: BINOMIAL THEOREM AND ITS SIMPLE APPLICATIONS
Unit 5 focuses on the Binomial Theorem for positive integral indices. It includes deriving and understanding the general term and middle term of a binomial expansion. The unit also covers simple applications of the theorem, providing insight into solving problems involving binomial expansions and their coefficients.
UNIT 6: SEQUENCE AND SERIES
Unit 6 delves into sequences and series, including Arithmetic Progressions (AP) and Geometric Progressions (GP). It addresses how to insert arithmetic and geometric means between two given numbers and explores the relationship between the Arithmetic Mean (A.M.) and the Geometric Mean (G.M.). This unit is essential for understanding patterns and series in mathematics.
UNIT 7: LIMIT, CONTINUITY, AND DIFFERENTIABILITY
Unit 7 covers essential concepts in limits, continuity, and differentiability. It begins with real-valued functions including polynomials, rational, trigonometric, logarithmic, and exponential functions, along with their inverse functions. The unit examines graphs of simple functions and foundational principles such as limits and continuity.
Differentiability is a key focus, with topics including the differentiation of sums, differences, products, and quotients of functions. It also covers differentiation techniques for trigonometric, inverse trigonometric, logarithmic, exponential, composite, and implicit functions, including derivatives of order up to two. Applications of derivatives are explored, such as understanding the rate of change of quantities, identifying monotonic functions, and finding maxima and minima of functions of one variable.
UNIT 8: INTEGRAL CALCULUS
Unit 8 focuses on Integral Calculus, beginning with integrals as anti-derivatives. It includes fundamental integration techniques for algebraic, trigonometric, exponential, and logarithmic functions. Key methods covered are integration by substitution, by parts, and by partial fractions, as well as using trigonometric identities for integration.
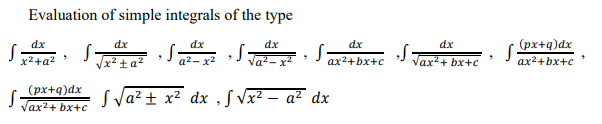
The unit also explores the Fundamental Theorem of Calculus and properties of definite integrals, which are crucial for evaluating integrals and understanding their applications. Students will learn to evaluate definite integrals and determine the areas of regions bounded by simple curves in standard forms. This section is essential for solving problems involving accumulation and area under curves.
UNIT 9: DIFFERENTIAL EQUATIONS
Unit 9 covers differential equations, focusing on ordinary differential equations (ODEs) and their characteristics, including order and degree. The unit explores methods for solving differential equations, starting with the separation of variables technique. It also addresses solutions for both homogeneous and linear differential equations, specifically those of the form:
dydx+p(x)y=q(x)\frac{dy}{dx} + p(x)y = q(x)dxdy+p(x)y=q(x)
This includes understanding how to find particular solutions and applying these methods to various types of differential equations.
Other JEE Main Related Syllabus Link
| JEE Main Syllabus | JEE Main Physics Syllabus |
| JEE Main Chemistry Syllabus | JEE Main Preparation |
| JEE Main Eligibility | JEE Main Exam Pattern |
UNIT 10: COORDINATE GEOMETRY
Unit 10 focuses on Coordinate Geometry, beginning with the Cartesian system of rectangular coordinates in a plane. It includes the distance formula, section formula, and the concept of locus and its equations. Key topics also cover the slope of a line, properties of parallel and perpendicular lines, and finding intercepts on the coordinate axes.
The unit examines straight lines, including various forms of line equations, intersection points, angles between lines, conditions for the concurrence of three lines, and the distance from a point to a line. It also covers geometric properties related to a triangle, such as finding the centroid, orthocentre, and circumcentre.
For circles and conic sections, the unit includes standard and general forms of circle equations, radius, and center, as well as finding the equation of a circle given the endpoints of a diameter. It addresses intersections between lines and circles and explores conic sections—parabolas, ellipses, and hyperbolas—providing their equations in standard forms.
UNIT 11: THREE-DIMENSIONAL GEOMETRY
Unit 11 covers Three-Dimensional Geometry, focusing on the coordinates of points in space. It includes calculating the distance between two points and applying the section formula in 3D. The unit explores direction ratios and direction cosines, as well as finding the angle between two intersecting lines. It also examines skew lines, determining the shortest distance between them and their equation, and provides methods for deriving the equations of lines in three dimensions.
UNIT 12: VECTOR ALGEBRA
Unit 12 introduces Vector Algebra, distinguishing between vectors and scalars. It covers the addition of vectors and their components in both two-dimensional and three-dimensional spaces. The unit also delves into scalar and vector products, focusing on their definitions, properties, and applications. This section is essential for understanding vector operations and their uses in various mathematical and physical contexts.
UNIT 13: STATISTICS AND PROBABILITY
Unit 13 focuses on Statistics and Probability. It covers measures of central tendency, including the calculation of mean, median, and mode for both grouped and ungrouped data. The unit also explores measures of dispersion such as standard deviation, variance, and mean deviation. In Probability, key topics include the probability of an event and the addition and multiplication theorems. It also covers Bayes’ theorem and the concept of a probability distribution for a random variable.
UNIT 14: TRIGONOMETRY
Unit 14 covers Trigonometry, focusing on trigonometric identities and functions. It includes the study of inverse trigonometric functions and their properties. The unit provides a detailed exploration of how these functions and identities are used to solve various trigonometric problems and equations.
Other JEE Main Related Syllabus Link
| JEE Main Syllabus | JEE Main Physics Syllabus |
| JEE Main Chemistry Syllabus | JEE Main Preparation |
| JEE Main Eligibility | JEE Main Exam Pattern |
JEE Main 2025 Mathematics Syllabus FAQs
What are the key topics covered in the JEE Main Mathematics syllabus for 2025?
The key topics include Sets, Relations, and Functions; Complex Numbers and Quadratic Equations; Matrices and Determinants; Permutations and Combinations; Binomial Theorem; Sequence and Series; Limit, Continuity, and Differentiability; Integral Calculus; Differential Equations; Coordinate Geometry; Three-Dimensional Geometry; Vector Algebra; Statistics and Probability; and Trigonometry.
How can I prepare for the Permutations and Combinations section of the JEE Main Mathematics exam?
Focus on understanding the fundamental principles of counting, and practice problems involving permutations and combinations. Study the formulas for P(n,r)P(n, r)P(n,r) and C(n,r)C(n, r)C(n,r), and apply them to solve a variety of problems.
What methods are used to solve Differential Equations in the JEE Main syllabus?
Methods include separation of variables, solving homogeneous and linear differential equations of the form dydx+p(x)y=q(x)\frac{dy}{dx} + p(x)y = q(x)dxdy+p(x)y=q(x), and understanding their order and degree.
What are the major formulas to remember for Integral Calculus?
Key formulas include integration by substitution, integration by parts, integration by partial fractions, and the Fundamental Theorem of Calculus. Practice evaluating definite integrals and determining areas bounded by curves.
How can I approach the Coordinate Geometry section?
Study the Cartesian system, distance formula, section formula, and properties of lines and circles. Practice solving problems involving equations of lines and conic sections (parabola, ellipse, hyperbola).
What is the importance of Trigonometric Identities in the JEE Main Mathematics exam?
Trigonometric identities are crucial for simplifying expressions and solving equations involving trigonometric functions. Understanding these identities helps in solving complex problems efficiently.
How do I tackle problems in the Complex Numbers and Quadratic Equations section?
Learn to represent complex numbers in the form a+iba + iba+ib, use the Argand diagram, and perform operations like addition and multiplication. Practice solving quadratic equations in both real and complex number systems.
What should I focus on in the Statistics and Probability section?
Concentrate on calculating measures of central tendency (mean, median, mode), measures of dispersion (standard deviation, variance), and understanding probability concepts including theorems and probability distributions.
How can I improve my understanding of Vector Algebra for the JEE Main?
Study the definitions and operations of vectors and scalars, practice vector addition, and understand scalar and vector products. Work on problems involving components of vectors in different dimensions.
What are the key concepts in Three-Dimensional Geometry that I should master?
Focus on coordinates of points in space, distance between points, section formula, direction ratios and cosines, angles between lines, and shortest distance between skew lines. Practice deriving equations of lines and understanding their spatial relationships.
If you have any queries related to JEE Main 2025 Mathematics Syllabus, you can ask us by leaving your questions below in the comment box.

As a dedicated Biology Science graduate, I’m passionate about sharing the latest updates in national and state entrance exams through my blog. I aim to keep aspiring students informed about exam trends, important dates, and changes in syllabi. With a keen interest in education, I strive to offer valuable insights for students navigating the competitive landscape of entrance examinations and admission tests. Stay updated with me.


